Click here to
go to the JPU200Y home page.
Click here to go to the UPSCALE
home page.
The End
Professor Jones had been working on time theory for many years.
"And I have found the key equation," he told his daughter one day. "Time is a field. This machine I have made can manipulate, even reverse, that field."
Pushing a button as he spoke, he said, "This should make time run backward run time make should this," said he, spoke he as button a pushing.
"Field that, reverse even, manipulate can made have I machine this. Field a is time." Day one daughter his told he, "Equation key the found have I and."
Years many for theory time on working been had Jones Professor.
-- F. Brown, Nightmares and Geezenstacks.
In class today, we finished our discussion of antimatter. In the Supplementary Notes the material that we discussed is in the sections:
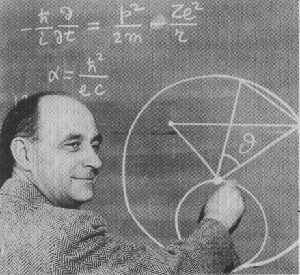ENRICO FERMI (1901-1954) was an Italian-born
physicist best known for his contributions to nuclear physics and the
development of quantum theory. In addition to his contributions to theory, he
was also noted as an experimentalist. Fermi was awarded the Nobel Prize for his
work on the nuclear process.
Fermi was famous for doing "back of the envelope" calculations in which the information given seems incomplete. Below I give you an example of solving a Fermi Problem. I then give you a list of other questions, and you will solve one of these. As usual, the assignment will be marked pass/fail.
As mentioned above, the assignment is due: In tutorial next week, March 14/15.
The problem we will solve is: How many piano tuners are in Toronto?
How might one figure out such a thing?? Surely the number of piano tuners in some way depends on the number of pianos. The number of pianos must connect in some way to the number of people in the area.
Approximately how many people are in the Greater Toronto Area?
4,000,000
Does every individual own a piano?
No
Would it be reasonable to assert that individuals don't tend to own
pianos; families do?
Yes
About how many families are there in Toronto?
Perhaps
1,000,000
Does every family own a piano?
No. Perhaps one out of every
five does. That would mean there are about 200,000 pianos in Toronto.
Do all of these need tuning?
No. Perhaps 1/2 of the pianos in Toronto are electronic and don't require tuning. Thus, there are about 100,000 pianos in Toronto that need tuning.
What about the pianos in the Conservatory of Music, Roy Thomson Hall, etc.?
Probably negligible compared to the 100,000 pianos owned privately.
How many pianos are tuned every year?
Some people never get
around to tuning their piano; some people tune their piano every month. If we
assume that "on the average" every piano gets tuned every two years, then there
are 50,000 "piano tunings" every year.
How many piano tunings can one piano tuner do?
Let's assume
that the average piano tuner can tune three pianos a day. Also assume that
there are 250 working days per year. That means that every tuner can tune about
750 pianos per year.
Finally, then how many piano tuners are needed in Toronto?
The number of tuners needed is about 50,000/750 = 67. Assuming we have just
enough tuners to satisfy the demand, there are therefore 67 piano tuners in
Toronto.
Using different assumptions for various factors, it is unlikely that you can justify an answer greater than a factor of 10 or smaller than a factor of 10 from the number we just obtained; that is to say, there are probably not more than 670 tuners and surely no less than 7. Thus the answer obtained is probably good to within an "order of magnitude."
The Yellow Pages list 19 entries under Pianos - Services, Supplies & Tuning. Some of these are one-person operations, but other, like the Canadian National Institute for the Blind and Paul Hahn & Co. almost certainly have more than one person who tunes pianos. There are also certainly other tuners not listed in this section of the Yellow Pages; for example the Piano Showcase explicitly says that they tune pianos, but are only listed in the Pianos section of the Yellow Pages.
Choose one of the following problems and solve it. Explain all the steps in your reasoning.
Note that Questions 2 and 3 will have some similar estimations in their solutions.
