The Concept of Energy

This little document discusses the development of the concept of energy in physics. Along the way, we will also discuss whether or not the energy is "real" or just a convenient way of thinking about the physical universe. Although almost no mathematics is used, some familiarity with classical mechanics at least at the high school level is assumed.

Vis Viva

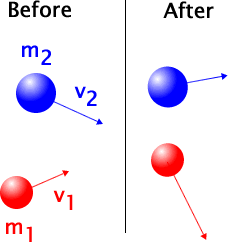
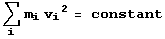
Consider a generic interaction between 2 objects, as shown to the right. A mass m1 moving with a speed v1 interacts with a mass m2 moving with a speed v2. Leibniz was a contemporary of Newton. He noticed that for many types of interactions the sum of the quantity mv2 was the same value before and after the interaction: He called this quantity the vis viva, or "living force," and for him its conservation was central to his thinking about interactions.

For Newton, more central than vis viva was the idea of momentum, a vector equal to the mass times the vector velocity. As you probably know, it is conserved in all interactions.

In the Newtonian description it turns out to be more convenient to talk about one-half of Leibniz' vis viva:

Now we call the quantity 1/2 m v² the kinetic energy, and we say that in some interactions the kinetic energy is conserved.

Other Forms of Energy

Above I was careful to say that in some interactions the kinetic energy is conserved. In other interactions, although the kinetic energy is not conserved we can construct the notion of a potential energy, and the sum of the kinetic and potential energy is conserved. In yet other interactions, we have to add yet another term: the heat energy of the objects. So we end up with three terms for the total energy: the kinetic energy plus the potential energy plus the heat energy, but still say that the total energy is conserved.

In time, even more terms were added: chemical energy, electrical energy, etc. etc. But the sum of all the terms remains constant.

An Analogy

Here is an analogy to the situation I have just described. As you probably know, from 1985 to 1995 a very popular comic strip was Calvin and Hobbes by Bill Watterson. In it a little boy named Calvin specialises in being bad. His best friend is a toy tiger named Hobbes, who only becomes alive when Calvin is present.

Let us imagine that Calvin has a collection of toy blocks, and every night after he goes to bed his parents pick up the blocks scattered all over the house and put them in the toy box. They notice that every night they end up with the same number of blocks. So they begin thinking about a concept of conservation of blocks.

One night after they have collected all the blocks they notice that they are 2 blocks short. But they look out the window and see 2 blocks in the back yard. So they now have 2 terms in their definition of the number of blocks:

But the principle, conservation of blocks, is still preserved.

A couple of weeks later the number of blocks in the toy box plus the number in the back yard is one less than the previous night. But they notice that Hobbes' stomach is a little distended. Of course they can't cut Hobbes open and see if he has swallowed a block. But they are clever (for parents) and weigh him. His weight has increased by the weight of one block. So now there is a third term in their calculation of the number of blocks:

Is the Energy Real?

This question can lead to deep philosophical discussions about the nature of reality and/or the way our minds think about it. We will not get nearly that deep in this section.

First, note that both our discussion of energy and the analogy to Calvin's blocks have used carefully defined measurement and calculational procedures. Such a procedure is called an operational definition, and using them tends to side-step questions of whether the object of the definition really exists or not.

For example, there are many arguments about the IQ of people and what if anything it really means. We can side-step those arguments by means of an operational definition:

I give sombody the Stanford-Binet IQ test. The person's IQ is defined to be the result of the test.

It is natural that when people begin thinking of some concept like conservation of energy and they find that every experimental test of the concept is consistent with the principle, they begin to think that the product of their thought really does correspond to some real object in the physical world. This belief can end up very strong.

For example, in radioactive beta decay, the observed decay products are an electron and a recoil nucleus. In the 1920's physicists began measuring the energies of the decay products and discovered that energy was not being conserved! But of course we must have our laws, so Pauli proposed in 1930 that there must be a third invisible decay product whose energy was not being measured. In 1933 Fermi named this particle a neutrino, which means "little neutral one" in Italian. Physicists were so convinced that the neutrino existed that 23 years later, in 1956, when it was experimentally discovered by Fred Reines and Clyde Cowan nobody got terribly excited: physicists' belief in conservation of energy was so strong that almost nobody doubted the existence of the neutrino.

We can sort of understand the kinetic energy: it is the energy objects have because of their motion. We can similarly sort of understand heat energy: it is the internal energy of vibration of the atoms and molecules. Similarly understanding the potential energy is more difficult: it is typically taught in introductory courses as the potential for work to be done on some object. However, it turns out that if we think about the fields that cause forces, the potential energy is just as real as all the other forms of energy, and is stored in the field.

Finally, in 1905 Einstein unified all the different forms of energy in what is probably the most famous equation in the world:

E = mc²

Acknowledgements

The Calvin and Hobbes story is similar to one told by Feynman in Richard P. Feynman, Robert B. Leighton and Matthew Sands, The Feynman Lectures on Physics, Volume 1, Chapter 4 (Addison-Wesley, 1963).

Author

This document was written by David M. Harrison, Department of Physics, University of Toronto, harrison@physics.utoronto.ca in April 2005.

This document is Copyright © 2005 David M. Harrison

This work is licensed under a Creative Commons License.