PHY357S:
Monday, 13 April 1998
Answer 1
(a) s-sbar, (ssc, anti-ssc), c-cbar, (s-bbar, b-sbar), (scb, anti-scb), b-bbar, (bbc, bbar-bbar-cbar). Brackets enclose hadrons of equal mass."sbar" indicates and anti-strange quark, and similarly for cbar & bbar.
(b) Mesons are made from quark-antiquark pairs. Since quarks have spin-1/2, there are two possible ways to make a meson with J=0: either the quark spins are antiparallel and they have zero orbital angular momentum (L=0), or the quark spins are antiparallel and they have L=1 in the opposite direction so the spin and orbitalal angular momentum cancel each other out. Since fermions and their antifermions have opposite intrinsic parity, the parity of a quark-antiquark pair is (-1)L+1. So in this case L=0 gives us P=-1 (pseudoscalar mesons), and L=1 gives us P=+1 (scalar mesons).
Answer 2
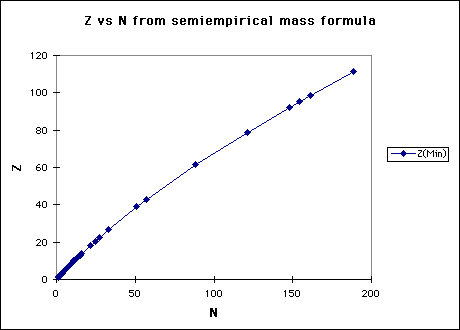
(a) The semiempirical mass formula (see F&H Eqn. 16.9)
has a minimum for fixed A when
so the most tightly bound nucleus will be for
(b) So we can see that as A increases, the ratio of neutrons to protons should increase.

(c) The nucleus will be stable if it has a smaller mass than the system produced under a, b-, b+, electron capture and fission decays. All beta decays just change Z without changing A, so by definition our value of Zmin would be stable against beta decays if not for the fact that the proton and neutron have slightly different masses. Looking at F&H Eq. 16.3 we can see that if neutron beta decays to a proton, it releases mn-(mp+me+mn)=0.78 MeV. This extra energy allows a number of beta decays, the lowest mass nuclei prediced to beta decay is A=20, Z=9.
For alpha decays, we calculate
and if it is negative the nucleus is predicted to be unstable. The mass formula predicts the nuclei are not stable against a decay if A>398.
For fission decays, we similarly calculate
The mass formula predicts the nuclei are not stable against a decay if A>92.
(d) From the Chart of nuclides we see that there is no stable isotope for A=5 and A=8, and the next value of A for which there is no stable isotope is A=210. Nuclei are more stable than our simple semi-empirical formula predicts.