Do not use the Back button of your browser when using MasteringPhysics.
"Be sure to make the [Mechanics] test real hard."
-- A PHY138 student from last year
There is a bug that can be triggered if you use the Back button on your browser. Under some circumstances the software will interpret this is submitting a previous wrong answer. The issue has been reported to the vendor. Meanwhile:
|
Do not use the Back button of your browser when using MasteringPhysics. |
|
The test is scheduled for Tuesday November 2 from 6 to 7:30 PM.
If you have a conflict with this time you must see Dr. Savaria in MP901A no later than 5PM this Friday, October 22.
We discussed an elastic collision between 2 objects of equal mass where initially one mass was moving and the other was stationary. Then, in the absence of spin, I proved that after the collision the angle between the 2 velocity vectors is Examples of such collisions are in playing billiards or in curling. The figure illustrates for a game of pool in which the player is trying to sink the 8 ball into the right-hand pocket. If from the point of collision the angle between the path to the right-hand pocket and the path to the left-hand pocket is 90 degrees, then if the 8 ball goes into the right-hand pocket the cue ball will go into the left-hand one. This is a "scratch" and causes the player to lose the game. Good players learn how to put spin on the cue ball to avoid the scratch. Beginning players should avoid trying to make such a shot. |
 |
| We used a Flash animation to illustrate the right-hand rule used to determine the direction of the vector angular velocity. The discussion included an introduction to the vector or cross product of 2 vectors, which is not discussed in the text until §10.5. We will return to this topic when we discuss that section of the text. |  |
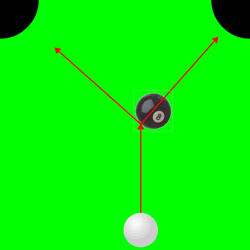Central to my approach to rotational motion is that all of the equations we have developed in our study of translational motion can be "re-cycled" into equations for rotational motion just by changing the symbols. Here is a table for the analogs. It is sort of similar to the text's Table 10.1 on page 318, but not the same.
Translational Quantity |
Rotational Equivalent |
|---|---|
r |
 |
v |
 |
a |
 |
m |
I |
For example, the translational kinetic energy is:
K = 1/2 m v2
Thus, we can immediately write the rotational kinetic energy by subsituting for m and v:
K = 1/2 I
A mistake in setting up the problem we were solving for elastic 2-dimensional collisions was discovered and corrected in purple. The mistake was corrected early Thursday morning, October 21. |
 |
 |
 |
/2 radians, or 90 degrees.