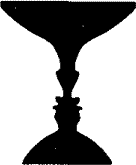AntiMatter
Click here to go
to the Physics Virtual Bookshelf
Click here to go to the UPSCALE
home page.
Introduction:
"The last level of metaphor in the Alice books [Alice in
Wonderland, Through the Looking Glass] is this: that life, viewed
rationally and without illusion, appears be be a nonsense tale told by an idiot
mathematician. At the heart of things, science finds only a mad never-ending
quadrille of Mock Turtle Waves and Gryphon particles. For a moment the waves
and particles dance in grotesque, inconceivably complex patterns capable of
reflecting their own absurdity. We all live slapstick lives ..." -- Martin
Gardner, The Annotated Alice.
"The universe is a practical joke of the general at the expense of the
particular." -- Aleister Crowley, The Book of Lies.
The Discovery of Antimatter
In 1932 there were three known "elementary particles:"
- electron
- proton
- neutron
Then Carl Anderson discovered a new one. He was working with a cloud
chamber, a forerunner to a bubble chamber.
We will illustrate Anderson's discovery by considering some bubble
chamber interactions. The bubble chamber is essentially a "tub" of superheated
liquid hydrogen. The hydrogen is not only the detector, but its protons form a
target for an incoming beam of particles. When a charged object goes through
the superheated hydrogen, it leaves behind a track of bubbles; for a cloud
chamber, such as used by Anderson, the track of a charged particle similarly
leaves behind a string of cloud droplets. In the bubble chamber experiments
below, the proton targets of the hydrogen are being bombarded with a beam of
high energy negatively charge pi mesons; these objects were, of course,
unknown to Anderson in 1932.
|
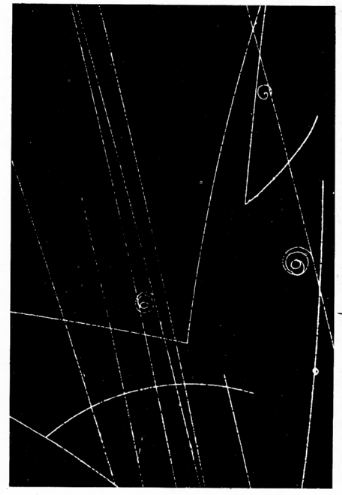
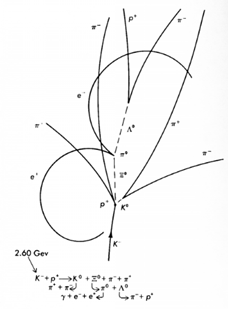
To the right we show a bubble chamber photograph. A number of
negatively charged pi mesons enters from the bottom; these are the beam
particles, and have a momentum of 1.20 Gev/c. The mesons are moving at 99.331%
of the speed of light.
One of the beam particles interacts with a proton to form a
neutral lambda and a neutral K; both of these leave no track in
the bubble chamber since they have no electric charge. Both of these neutral
particles then decay into charged particles, which do leave tracks in the
chamber. The lambda decays into a proton and a negatively charged pi meson; the
K decays into the positively charged pi meson and a negatively charged pi
meson. |
 |
|
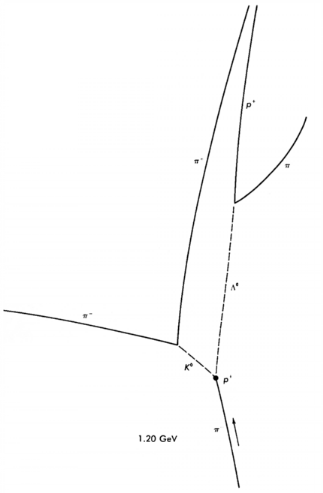
The diagram to the right shows a reconstruction of the interaction
we see in the photograph. |
 |
|
The reason for the curvature of the tracks is that there is a
magnetic field directed into the plane of the photograph. The figure to the
right shows the curvatures due to positive and negatively charged objects. Note
that for positively and negatively charged particles moving from the left to
right, their curvatures are in opposite directions. It will turn out to be
useful later to note that the curvature of a positively charged object moving
from right to left is identical to the curvature of the negative object moving
from left to right. By measuring the radius of curvature of a track in a bubble
chamber we can determine the momentum of the particle.
The faster the particle is moving, the less curvature there is in
its trajectory. Thus, the beam particles in the above bubble chamber photograph
are almost straight because they are moving at almost the speed of the
light. |
|
The new particle that Anderson discovered has the following
properties:
- The mass equals the mass of the electron.
- The charge has the same magnitude as the electron's charge, but is
positive instead of negative.
- The particle is always created in pairs with electrons.
- When this particle collides with an electron, both annihilate.
|
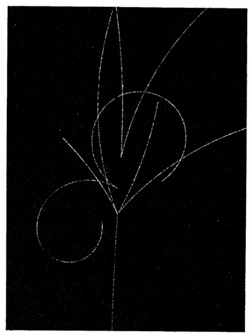
The figure to the right shows another bubble chamber photograph,
this time in which "pair production" occurred. The beam is a 2.60 GeV/c
negative K meson, again entering from the bottom. |
 |
|
In the reconstruction of the event, shown to the right, the
electron is labelled e- and the new particle that Anderson
discovered is labelled e+. We call this new particle the
antimatter electron or positron. It is now known that all
so-called elementary particles have antimatter counterparts.
All the bubble chamber photographs and
reconstructions of the events are from Harvey E. White, Modern College
Physics 6th ed. (Van Nostrand Reinhold, 1972), pg 1011 and
1014. |
 |
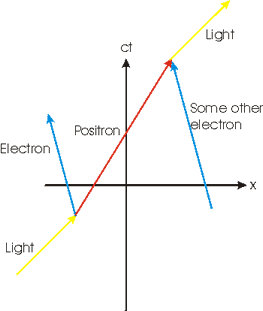
| The figure to the right is the spacetime diagram for pair production and subsequent annihilation of the positron. Light energy comes in from the lower left. It creates a positron-electron pair; this is a conversion of the light energy into mass energy. The positron goes along until it collides with some other electron, which causes both to annihilate; the mass energy is converted into light energy. |
 |
When the positron was discovered, Heisenberg was particularly elated. It was
already known that the neutron was unstable, decaying into a proton and an electron.
This naturally led some people to think of a neutron as being made of a proton
and electron. With the discovery of the positron, Heisenberg could just as easily
think of the proton as being made of a neutron plus a positron. As he later
wrote: "The symmetry between the proton and neutron was restored ... In the
beginning was symmetry!" (Reference: Physics and Beyond, pg. 132.) In
this view the proton and neutron are made of each other. This is an example
of what we shall later be calling a bootstrap.
A simple Flash animation of pair production and annihilation may be found here.
The animation will appear in a separate window. It requires Version 5 or better
of the Flash player, which is available free from http://www.macromedia.com/.
The file size if 4.7k.
Dirac's Theory of Antimatter
A comment by Dirac on why the positron was not discovered until
1932:
"Why did the experimentalists not see them? Because they were prejudiced
against them. The experimentalists had been doing lots of experiments where
particles were moving along curved tracks in a magnetic field. .... [They]
sometimes saw the opposite curvature, and interpreted the tracks as electrons
which happened to be moving into the source, instead of the positively charged
particles coming out. That was the general feeling. People were so prejudiced
against new particles that they never examined the statistics of these
particles entering the source to see that there were really too many of them."
-- Dirac, in J. Mehra, ed. The Physicist's Conception of Nature, pg. 12.
Note that Dirac is referring above to the fact we noted above that a
negatively charged object moving from left to right in a magnetic field has the
same curvature as a positively charged object moving from right to left.
If Dirac sounds a bit grumpy in the above quotation, there is a reason.
He almost predicted the existence of the positron in 1930, before Anderson's
discovery. He was musing about why the energy is always a positive number. He
could think of no reason why this should be so. Applying a common physicists'
assumption, that what is not forbidden is required, he concluded that there
must be negative energy objects like electrons.
The next question, then, is why don't we observe these negative energy
electrons. Dirac concluded that it must be because there is an infinitely dense
totally homogeneous sea of these electrons everywhere in the universe. And
since it is homogeneous it is unobservable.
Inayat Khan tells a Hindu story that has some relevance to this
unobservable sea. A fish went to the Queen fish and asked: "I have always heard
about the sea, but what is the sea? Where is it?" The Queen fish replied: "You
live, move, and have your being in the sea. The sea is within you and without
you, and you are made of sea and you will end in sea. The sea surrounds you as
your own being." Reference: P. Reps, Zen Flesh, Zen Bones pg. 211.
Another example of a homogeneous and therefore unobservable structure is
the now superfluous concept of an all-pervading luminiferous ether as a medium
for light. Because this ether was homogeneous we could not detect it.
Now imagine that some light energy collides with a negative energy
electron in Dirac's sea, knocking it into a positive energy state. This
electron is observable. The hole it leaves in the sea is also observable,
since it differs from the homogeneous negative energy sea around it. In
fact, the hole will appear to have the mass of an electron. Further, its
charge will appear to have the magnitude of the charge of the electron but
will be positive. In fact, in Dirac's theory the hole is the positron!
When Dirac got this far, the positron was unknown. Thus he tried to
interpret the hole as being a proton, but the mathematics of this theory didn't
quite work out. When Anderson discovered the positron, Dirac immediately
realised his theory described it perfectly. This theory of the positron is
still routinely
used.
|
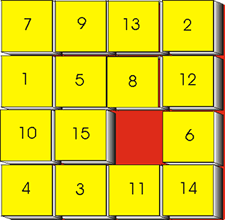
The puzzle to the right was invented by Sam Loyd. The object of
the puzzle is to re-arrange the tiles so that they are in numerical order.
The puzzle forms a model of how the positron moves in Dirac's
theory. The numbered tiles represent the negative-energy electrons. The hole is
the positron. When a negative-energy electron falls into the hole, the hole
appears to have moved to another position. |
 |
What appears to be the annihilation of the positron when it collides
with some other electron, then, is just the electron falling into the hole,
giving up its energy as light.
Feynman's Theory of Antimatter
In 1949 Richard Feynman
devised another theory of antimatter.
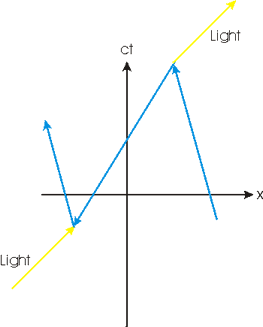
The spacetime diagram for pair production and annihilation
appears to the right. An electron is travelling along from the lower
right, interacts with some light energy and starts travelling backwards
in time. An electron travelling backwards in time is what we call
a positron. In the diagram, the electron travelling backwards in
time interacts with some other light energy and starts travelling
forwards in time again. Note that throughout, there is only one
electron.
A friend of mine finds the image of an electron travelling backwards
in time, interpreted by us as a positron, to be scary.
Feynman in his original paper proposing this theory wrote:
"It is as though a bombardier flying low over a road suddenly sees
three roads and it is only when two of them come together and disappear
again that he realizes that he has simply passed over a long switchback
in a single road." (Physical Review 76, (1949), 749.) |
 |
Note that Feynman's theory is yet another echo of the fact, noted above,
that a negatively charged object moving from left to right in a magnetic field
has the same curvature as a positive object moving from right to left.
Feynman's theory is mathematically equivalent to Dirac's, although the
interpretations are quite different. Which formalism a physicist uses when
dealing with antimatter is usually a matter of which form has the simplest
structure for the particular problem being solved.
Note that in Feynman's theory, there is no pair production or
annihilation. Instead the electron is just interacting with electromagnetic
radiation, i.e. light. Thus the whole process is just another aspect of the
fact that accelerating electric charges radiate electric and magnetic fields;
here the radiation process is sufficiently violent to reverse the direction of
the electron's travel in time.
Nambu commented on Feynman's theory in 1950:
"The time itself loses sense as the indicator of the development of
phenomena; there are particles which flow down as well as up the stream of
time; the eventual creation and annihilation of pairs that may occur now and
then is no creation or annihilation, but only a change of direction of moving
particles, from past to future, or from future to past." (Progress in
Theoretical Physics 5, (1950) 82).
About Formally Equivalent Descriptions
Above we noted that Feynman's theory of antimatter is formally
equivalent to Dirac's theory. This means that the mathematics of either
theory can be manipulated into the other one. Here is a nearly trivial
example to illustrate. We say that there are two variables that describe
some physical property: y and t. Perhaps y is the position
of some object being acted on by various forces, and t is
the time.
We
imagine that some theory gives the relation between the two variables
as:
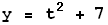
Then we "read" the mathematics of the theory as a statement that tells us
what the position of the object is for various times.
We say that t is
the
independent variable, and that y is dependent on x.
Here are some values:
| Independent Variable |
Dependent Variable |
| 1 |
8 |
| 2 |
11 |
| 3 |
16 |
Some other theory relates y and t according to:
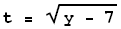
Now we "read" the mathematics as a statement that says that for a given
position of the object we can calculate when it was at that position.
We say that now y is the independent various and t the
dependent variable.
| Independent Variable |
Dependent Variable |
| 8 |
1 |
| 11 |
2 |
| 16 |
3 |
But the two equations are mathematically and formally equivalent to each
other. Thus although the way we view whatever process is being described
differently depending on which equation is being used, the underlying formalism
is the same.
When we return to Quantum Mechanics, we will see another example of two
formally equivalent theories. These are the Heisenberg form of Quantum
Mechanics and the Schrödinger one. There we shall see that despite the
formal equivalence, both Heisenberg and Schrödinger felt strongly about
which one is "correct."
A Representation of Both Theories of Antimatter
| "Day and Night" by M.C. Escher. Note that both Dirac's
and Feynman's theory of antimatter are represented. This was not Escher's
intention, of course. Instead, in this and much of his work he was
focusing on figure-ground studies. |
 |
Thousands of years ago Chuang Tsu wrote a commentary that seems apropos
for both Escher's drawing and these two theories of antimatter:
"It comes out from no source, it goes back in through no aperture. It
has reality yet no place where it resides; it has duration yet no beginning or
end. Something emerges, though through no aperture - this refers to the fact
that it has reality. It has reality yet there is no place where it resides -
this refers to the dimension of space. It has duration but no beginning or end
- this refers to the dimension of time. There is life, there is death, there is
a coming out, there is a going back in - yet in the coming out and going back
its form is never seen." (23, Watson trans.)
| The figure to the right is a classic figure-ground study.
Looked at one way the figure is a vase; from another perspective it is two
faces. The distinction between the figure and the ground defining the figure is
reminiscent for me of Dirac's infinite homogeneous sea of negative energy
electrons. |
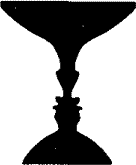 |
Gestalt psychology uses figures like these. Some people have difficulty
in seeing one or the other of the images, and these psychologists have devised
techniques to aid people in learning to see both and switch back and forth
between them. Of course, their goal in this is quite a bit more than just
seeing both images in a figure-ground study.
Although most people can switch back and forth between the two ways of
seeing the above figure, we can not see both at once. This reminds of so-called
wave-particle duality for electrons: we can think of the electron as a wave or
as a particle but can't think of it as both at once. The actual figure, though,
is both the vase and the faces; similarly the electron is both a
wave and a particle.
Why Is This Topic In The SYMMETRY Part of the Syllabus?
Recall that in the very early days of the universe, matter was created
through pair production of electrons-positrons and protons-anti-protons. Does
this mean that one-half of the matter in the universe is antimatter? Almost all
experts in this field know the answer to the question. The problem is that some
of the experts think the answer is "Yes" and others think the answer is
"No."
Recall that a simple model of a hydrogen atom is a positively charged
proton with a negatively charged electron in orbit around it. We can similarly
have an anti-hydrogen atom with a negatively charged antiproton with a
positively charged positron in orbit around it. This anti-hydrogen atom has been experimentally produced.
Similarly, we can have
anti-helium, anti-molecules, etc.
It is certainly possible that there are antimatter galaxies, with
anti-solar systems and maybe anti-people. In Dirac's theory we might say that
the anti-people are holes in an infinite sea of negative energy people. They,
of course, would say that we are holes in an infinite sea of negative energy
anti-people.
Imagine that we get in radio contact with intelligent aliens from, say,
the Planet of the Apes. With painstaking effort we establish a common language
and end up in a state of good communication. One of the things that we want to
communicate is the difference between left and right. It turns
out that the only way to do this is to use the violation of mirror symmetry by
weak interactions. So, we can have the aliens construct an apparatus like
Madame Wu used in her classic experiment, and with that it is possible to
define right and left.
However, if they are in an antimatter part of the universe, their
anti-Madame Wu apparatus will get the definition backwards. So, if we set up a
face to face meeting with the aliens and, having told them about some of our
customs, when we meet they want to shake hands with us and extend their left
hand, we better run!
Before the 1950's the validity of mirror symmetry was considered to be a
nearly self-evident truth; you may recall that this symmetry is sometimes
called conservation of parity. Yet another symmetry that used to be
believed to be true is called charge conjugation: if we turn all
particles into their anti-particles (and all anti-particles into their
particles) everything will work the same. Now we know that neither parity or
charge conjugation are completely conserved. But from the anti-Madame Wu
experiments we can say that together they are conserved. Turn all particles
into their anti-particles and then turn the whole system into its mirror image;
the result will work the same. We shall have more to say about this in the next
section of the syllabus titled Time's Arrow.
Symmetry and Conservation
In the previous section we sometimes talked about a symmetry, such as
mirror symmetry, and sometimes describe the same circumstance in terms of a
conservation of some quantity. There turns out to be a very close connection
between symmetry and conservation.
For every symmetry, there is a corresponding conservation. The
converse is also true: for every conservation, there is a corresponding
symmetry.
For example, symmetry under spatial translation is equivalent to
conservation of momentum. Symmetry under time translation is equivalent to
conservation of energy. And symmetry under rotations is equivalent to
conservation of angular momentum
Charge Conjugation, Parity, and Time Reversal
Above we indicated that in weak interactions such as used in Madame Wu's
experiment, if we take the apparatus and construct a mirror image of it, and
then take all the particles of the apparatus and turn them into their
corresponding antiparticles, this new apparatus will work exactly like the
original one. Sometimes, this is called CP Invariance, where the
C stands for charge conjugation and the P for parity ie. mirror
reflection.
There is a meson called the K 0, which is electrically
neutral and decays via the weak interaction into either:
- an electron, a positive pi meson and an anti-neutrino; or
- a positron, a negative pi meson, and a neutrino.
It turns out that the second mode is slightly preferred over the
first.
The K 0 has an antimatter pair, the K
bar0, which also decays via these same two modes, and for the
anti-K meson the second mode is also slightly preferred over the first.
If one draws pictures of these decays, including the helicity states of
the decay products, it isn't too difficult to realise that these experimental
facts means that CP Invariance is violated.
However, according to our best understanding, if we:
- Turn all particles into their antiparticles (C); and
- Subject the decays to a mirror reflection (P); and
- Reverse the direction of time (T)
the combination of these three operations leaves the decay invariant.
This is sometimes called CPT Invariance.
We summarise the invariances and symmetries that we have been
discussing:
- Space translation, time translation, rotation: believed to be
absolutely true.
- Mirror reflection (P): true for gravitational, electromagnetic, and
strong interactions; not true for weak interactions.
- Turning particles into antiparticles + turning antiparticles into
particles (C) + P: true for all interactions except for a small subset of weak
interactions.
- C + P + reversing the direction of time (T): believed to be
absolutely true.
A Great Question From a Former Student
Is the existence of symmetry due to the existence of non-symmetry?
A Final Speculation
|
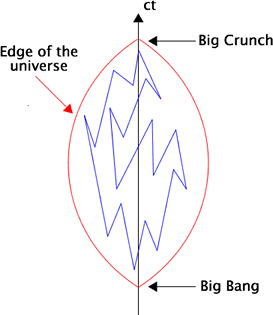
Wheeler once called Feynman in the middle of the night, saying
"There is only one electron in the universe!" The figure to the right shows
what he meant. We assume the universe is closed. In Feynman's theory of
antimatter, all the worldlines of the electrons and positrons connect so
throughout there is only the one electron. This "explains" a mysterious fact:
all electrons have totally identical properties. |
 |
Author
This document was written by David M. Harrison, Dept. of Physics, Univ. of Toronto, harrison@physics.utoronto.ca in 1999.
This is $Revision: 1.11 $, $Date: 2006/03/22 16:10:41 $ (y/m/d UTC).
This document is Copyright © 1999 - 2005 by David M. Harrison.