Conservation Laws

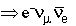
Particle  (mass)JPC  Þdecay	Mean Lifetime (t)	Decay Width	Decay Fraction	Comments / Conservation Laws
r0 (769)1--	4x10-24s	151 MeV
Þ p+p-		150 MeV	99%
Þ p+p-g		1.5 MeV	1%	~a
Þ e+e-		7 KeV	4.5x10-5	~a2 EM (electromagnetic)
Þ g				momentum
Þ p0p0				Bose-Einstein (BE) statistics
Þ gg				Landau-Yang theorem (BE, J)
p0 (135)0-+	8x10-17s	8 eV		lightest hadron
Þ gg		8 eV	98.8%	~a2 EM
Þ e+e-g		9x10-2eV	1.2%	~a3 EM
Þ e+e-e+e-		2x10-4eV	3x10-5	~a4 EM
Þ e+e-		6x10-7eV	8x10-8	~a4 EM (Note)
Þ ggg		2x10-7eV	<3x10-8	~a3 (C)
D0(1232)3/2+	5x10-24s	120 MeV
Þ Np (pp- or np0)		120 MeV	>99%
N0(1440)1/2+	2x10-24s	350 MeV
Þ Np		220 MeV	65%
Þ Npp		120 MeV	35%
n (940)1/2+	887s	7x10-25eV
Þ pp-				energy
Þ pe-				J (angular momentum) & L
Þ p+e-		<2 x 10-55eV		Baryon number (B) & lepton number (L)
		7x10-25eV	~100%	weak
p (938)1/2+	>1025y	<10-54eV		lightest baryon
Þ p0e+	>1033y	<10-62eV		B & L
d (np)1+	>1021y?
Þ p0r+	>1029y?			B
p- Þ e-ne		?		L
L (1115)	2.6x10-10s	3x10-12eV		weak
Þ Np		3x10-12eV	100%	strangeness (S)
K+ (494)0-	1.2x10-8s			weak
Þp+p0			21.2%	S
Þ m+nm			63.5%	S
m- (106)1/2	2.2ms	3x10-16eV
Þ e-g		<10-26eV	<5x10-11	muon number (Lm) and electron number (Le)
		3x10-16eV	~100%	weak
e- (0.511)1/2	>1023y
Þ neg	>1025y			electric charge (Q)
h (547)	6x10-19s	1.2 KeV
Þp+p-		<2 eV	<0.15%	Parity (P) & CP
Þp+p-p0		0.3 KeV	23%	EM (G-parity: isospin(I)+C)
Þgg		0.5 KeV	39%	electromagnetic (EM)
p0Þ ggg		2x10-7eV	<3x10-8	~a3 charge conjugation (C)
p+ (140)0-	2.6x10-8s	2x10-8eV		lightest charged hadron and charged boson
Þ m+nm		2x10-8eV	99.98%	weak
Þ e+ne		3x10-12eV	1.2x10-4	helicity
K0L (498)0-	5x10-8s	1x10-8 eV
Þp+p-		3x10-11 eV	0.2%	CP
Þp+ p- p0		2x10-9 eV	12.6%

Notes:

Decay Width : Because of Heisenberg uncertainty principle, any particle with a finite lifetime has a mass distribution of non-zero width. The total decay width (G=h/2pt) is the Full Width at Half Maximum of the mass distribution. Each decay channel contributes to the total width, and the partial decay width (G_i = G · BR) is the contribution to the total width due to a specific final state. (Return)

Decay Fraction : Decay branching fraction or branching ratio (BR) is the probability of a particle decaying into a specific final state. The sum of the branching ratios should add up to 100%. (Sometimes "branching ratio" refers to a ratio of branching fractions, but in particle physics "branching ratio" is usually synonymous with "branching fraction".) (Return)

p⁰ Þ e⁺e^- : Mediated by 2 virtual photons, not 1, since the pion has spin-0. The decay is also suppressed by an helicity factor of (m_e/m_p)². ( Return)

Helicity : Helicity is the normalized projection of a particle's spin on to its momentum, i.e. J·p/J·p. Helicity is not a relativisticly invariant quantity since momentum is frame dependent, but helicity is conserved to the extent that the probability of the helicity remaining the same is typically b=v/c. (Note: This is true independent of whether parity is conserverd.) (Return)

Flavour Conservation Laws

Strangeness (S), muon number (L_m), and electron number (L_e) are examples of a "flavour" quantum numbers. These conservation laws are simply a reflection of the existence of the fundamental fermions.

The strong and electromagnetic interactions conserve the fermion flavours, e.g. the only way to get rid of an electron is to annihilate it with an anti-electron (a positron). Similarly, the only way for strong or electromagnetic interactions to get rid of a strange quark is to annihilate it with an anti-strange quark. In this sense we actually have 12 conservation laws, but some are of little importance (i.e. neutrinos don't interact strongly or electromagnetically) or are obscured by other symmetries.

Weak interactions can transform any "up" type quark (u, c, or t) into any "down" type quark (d, s, b), and vice versa, but "up" or "down" type quarks don't mix among themselves. The relative probabilities depend on the transistion. Weak interactions may also not conserve lepton flavours in a similar manner, but the experimental evidence for such transitions is not yet compelling.

Isospin

Isospin symmetry is invariance under the interchange of u and d quarks. In nuclear physics, this corresponds to invariance under the interchange of protons and neutrons. In analogy to the two spin states of a fermion, up quarks (or protons) are considered to be the I₃=+¹/₂ state, and down quarks (or neutrons) are considered to the the I₃=-¹/₂ state. Mesons (quark-antiquark hadrons) made from u & d quarks can have isospin 0 or 1; u & d quark baryons (qqq states) can have isopin ¹/₂ or ³/₂; nuclei can have I=0, ¹/₂, 1, ³/₂ …

This symmetry is because u and d quarks have negligible mass compared to their strong binding energies in any hadron. As a result, replacing an up quark with a down quark makes almost no difference. For example, a proton (uud) is essentially the same as a neutron (udd), except for a tiny mass difference (~0.1%) and their electric charges.

Isospin symmetry is an accident, since there is no obvious reason why the up and down quarks should have the masses they do, but it is very powerful in understanding hadronic systems and their interactions. The symmetry is not exact, since u and d quarks do have slightly different masses and very different electric charges.

Isospin (I) is conserved by strong interactions. I₃ is conserved by both strong and electromagnetic interactions, since the net number of u quarks and and the net number d quarks never changes. Isospin is a mixture of u & d flavour symmetry with the consequences of u & d quark mass degeneracy.

Sometimes both isospin invariance and charge conjugation invariance cannot both be true; this consistancy can be parameterized using the G-parity quantum number. G corresponds to making a parity transformation in isospin space giving a factor (-1)^I, followed by a C transformation. G is conserved by strong interactions, since both I and C are conserved, but not by weak or electromagnetic interactions.

Parity

There are two obvious space transformations: translation (xÆx+dx) and reflection (xÆ-x). Translation is a continuous operation, but reflection is discrete - it is impossible to reflect by an infinitesimal amount.

The reflection operation is known as the parity operator, i.e.

In general, the parity operator reverses all 3-vectors, e.g.

but not axial-vectors, e.g.

Spherical harmonics (F&H Table A8) are the eigenfunctions of the orbital angular momentum operators and have parity

The relative parity of particles and antiparticles can be determined from the form of their relativistic wave functions. Particles and antiparticles have the same parity if they are bosons; particles and antiparticles have opposite parity if they are fermions.

If y(x) is an eigenfunction of parity, then

where the eigenvalues of the observable are P=±1 because

If parity is conserved, then the parity of a system is the product of the parity of its components.

In general,discrete symmetries lead to multiplicative conserved quantitites.

Strong and electromagnetic interactions conserve P (e.g. hÞp⁺p^- is forbidden); weak interactions do not conserve P.

The Tau-Theta Puzzle

In the early 1950's, two strange particle decays were observed

Pions are eigenstates of parity with intrinsic spin^Parity

The parity of two pions (p₁ & p₂) with relative orbital angular momentum L(1,2) is

P(pp)	= P(p1) x P(p2) x (-1)L(1,2)
	= (-1) x (-1) x (-1)L(1,2)
	= (-1)L(1,2)

The parity of three pions is

P(ppp)	= [P(p1) x P(p2) x (-1)L(1,2)] x P(p3) x (-1)L(12,3)
	= - (-1)L(1,2) x (-1)L(12,3)
	= - (-1)L(1,2)+ L(12,3)

where L(12,3) is the orbital angular momentum between the third pion and the first two. If J is the spin of the parent particle, then by angular momentum conservation, we must have

If parity is conserved in the decay, the parity of the parent particle is the same as the parity of the final state pion system. So we expect

So the q⁺ and t⁺ cannot have the same spin^parity (J^P) if parity is conserved, and the q⁺ and t⁺ must be different particles.

The existence of two new particles would not be of concern except that the q⁺ and t⁺ appeared to be otherwise identical - they had the same masses, the same lifetimes, and were produced and interacted with the same lifetimes.

Further measurements showed that J(q⁺) = J(t⁺) = 0.

Why would there be two identical particles differing only by their parity?

In 1956 T. D. Lee and C. N. Yang pointed out that parity conservation had never been tested for the weak interaction. If parity was not conserved, the q⁺ and t⁺ were simply the three and two pion decay modes of a single particle.

C.S. Wu quickly tested parity conservation in beta decays of Co⁶⁰ and discovered that parity is 100% violated. Neutrinos are "left-handed", their spin is always anti-parallel with their momentum.

These results were immediately confirmed by other experiments. These, or similar experiments, could have been done 30 years earlier. (In fact, some experiments probably saw parity violating effects in the 1920's, but nobody realized it since nobody expected it. Part of the problem was that the idea and importance of "parity" was only recognized in the 1930's by Wigner.)

The q⁺/t⁺ is now known as the K⁺.

Charge Conjugation

Charge conjugation reverses all "charges", e.g electric charge, baryon number, lepton number, strangeness, muon number, ….

Cy =

Positions, energy, momenta, angular momenta, and spins are not affected by the C operator.

If y is an eigenstate of of charge conjugation, then

where the eigenvalues of the observable are P_C=±1 because

C²y = CP_C = P_CC = P_C²y

A wavefunction may be in an eigenstate of C

if all additive quantum numbers are zero. Electromagnetic fields are produced by charges which change sign under charge conjugation, so

The C parity of neutral fermion-antifermion systems can be determined either by experiment, or by more detailed relativistic quantum analysis giving C=(-1)^L+S.

(Where the particle symbol represents the "charge" part of the particle wavefunction.) Particles which are not their own antiparticles cannot be eigenstates of C, e.g.

If charge conjugation is a symmetry of the physics, then the charge conjugation eigenvalue is a multiplicative conserved quantity. Strong and electromagnetic interactions conserve C (e.g. p⁰Þggg is forbidden); weak interactions do not conserve C.

CP

CP is the combined charge conjugation - parity operator. The weak interaction violates C and P a 100%, but CP is almost conserved. The only place this CP violation has yet been observed is in the the nutral kaon system.

Strangeness eigenstates:

CP eigenstates:

Mass eigenstates:

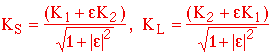
K₂Þppp is allowed, but K₂Þpp is forbidden by CP invariance. The K_L ("K-long") is mostly K₂ and has a much longer lifetime than the K_S ("K-short") because there is so little phase space for KÞppp decays relative to K₂Þpp decays.