Introduction to
Boyle's Law

In this document we introduce the Boyle's Law experiment from the Physics laboratory at the University of Toronto. It is intended to be used before you begin the experiment. The Preparatory Questions at the end of this document should be answered and turned in to your Demonstrator before you begin to work on the experiment.

Requirements

In order to effectively use this page, your browser needs to be capable of viewing Flash animations, also known as swf files. The Flash player is available free from http://www.macromedia.com/. The animations are for Version 5 of the player or later.

You will also want to have either the Real Media or QuickTime video player installed on your computer. The Real Media player is available free from http://www.realnetworks.com/. The QuickTime player is available free from http://www.apple.com/quicktime/.

A document of the soundtrack of the video and a summary of the information in this page are also available below. They are in pdf format. Accessing these documents will require that you have the Acrobat Reader, which is available free from http://www.adobe.com

Introduction

Robert Boyle and others formulated a law relating the pressure and volume of a constant quantity of gas at constant temperature. The basis of the law was first published by Boyle in 1662.

What today we call Boyle's Law was the work of more than one person. It was originally an unexplained experimental fact. Much later, theoretical physicists derived the relationship using the kinetic theory of gases. You study theoretical physics in the lecture component of your course. In this laboratory you are learning about the other "better half" of physics: experimental physics.

The Apparatus

We have prepared a video in various formats to introduce you to the apparatus; the running time of the video is almost exactly 3:00 minutes. Links to the video appear below. You will wish to adjust the volume of the speakers on your computer so that you can easily hear the soundtrack. For the higher resolution RealMedia version, you may also wish to increase the size of the video using the controls provided by the player.

You may download a pdf version of the soundtrack of the video by clicking on the red button to the right. It will appear in a separate window, and has a file size of 73k.

The Streaming video will be played by the RealMedia player as it is delivered to your computer by the network. The Download versions of the video will be downloaded to a temporary area on your local hard disc and then shown if your browser is configured to use the appropriate player. You may save these Download versions to a more permanent place that you specify on your computer's discs by right clicking on the link and then saving the Target (Internet Explorer) or Link (Netscape).

RealMedia Streaming
RealMedia Download	Less Resolution (746k): Click here More Resolution (1.7M): Click here
Quicktime Download	Less Resolution (893k): Click here More Resolution (2.0M): Click here

We have prepared a diagram of the apparatus. Click on the yellow button to the right to see it. It will appear in a separate window. The size of the graphic file is 32k.

There is a Flash animation of the effect of changing the height of the mercury reservoir on the heights of the mercury in the tubes. It may be accessed by clicking on the figure to the right: it will appear in a separate window. The size of the animation file is 13k.

Data Collection

For this experiment you need to understand how manometers work. The apparatus for the experiment is a manometer; in addition you will use a barometer, another manometer, to measure atmospheric pressure.

A small document on manometers may be accessed by clicking the blue button on the right. It will appear in a separate window.

You will need to know atmospheric pressure for this experiment. It is possible that the value will change during the course of your data collection, so you should measure it just before you begin taking data and immediately after you are finished. You should also record the temperature of the room before and after taking data.

You will measure atmospheric pressure with a barometer. There are barometers mounted on the central pillars in both MP125 and MP126. You may access a document on using a barometer by clicking on the green button to the right. It will appear in a separate window.

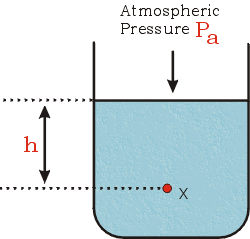
The figure to the right shows the tubes and Mercury columns for a particular position of the reservoir. The pressure of the gas in the closed end of the tube is related to the length h and to atmospheric pressure. The barometer measures atmospheric pressure in units of mm of Mercury (Torr). For most of your data analysis, expressing h in mm so that the pressure of the gas is in Torr will be appropriate. If atmospheric pressure is Pa then the pressure of the gas is: (Pa + h) for the case shown to the right. (Pa - h) if the point A is lower than the point C. The volume of the gas is related to the length L and the cross-sectional area of the tube. For most of your data analysis, you may measure the volume in units of the length of the tube.

You should take data for as wide a range of values of pressure and volume as the apparatus allows.

Do not move the reservoir up or down too quickly. This can cause the mercury to overshoot and cause a spill. As discussed below, after moving the reservoir you should wait a few seconds before taking readings.

Data Analysis

Verifying Boyle's Law

Since

p V = constant

p = constant / V

Thus, fitting p versus 1/V to a straight line should give a good fit with an intercept equal to zero within errors.

However, a systematic error in the volume is possible because the top of the column of air in the tube is not well defined. This can give a poor fit to the above relation.

Another useful fit is to pV versus 1/V to a straight line. One of the nice things about this fit is that even in the presence of a small systematic error in the volume, the values of pV are almost the same value for all the datapoints, so the graph will use an expanded scale and the error bars can be easily seen.

If Boyle's Law is true for your data, then this fit should yield a straight line with a slope equal to zero within errors. If this is not the case, it is likely that the systematic effect in the volume measurement is responsible. However this effect, due to a small mis-identification of the position of the effective top of the closed end of the tube, should be negligible for infinite volume. Infinite volume corresponds to a zero value of one over the volume, so the intercept of your straight line fit should correspond to the true value of pV. From this number, you can determine the value of your systematic error, if any, and correct your volume data.

Finally, is Boyle's Law true for your sample of gas?

If yes, calculate a final value of pV and its error.

If no, why have most experiments over the past 350 years concluded that it was true?

Converting to Energy Units

The SI unit of pressure is the pascal, which is newtons per square meter.

The SI unit of volume is, of course, meters³.

Thus pV has units of newton meters, which is the unit of energy, the joule. Now you will convert your final value of pV, including its error, to joules.

So far you have probably been measuring volume in units of the length of the tube. To convert to SI units you will need to know that the manufacturer of the glass tubing states that its cross-sectional area is:

A = 0.1225 ± 0.0005 cm²

To convert pressure from mm of Mercury to pascals requires knowing the values of the acceleration due to gravity, g, and the density of Mercury. This conversion is discussed in most textbooks, and also in the web document on manometers accessed above.

However depends on temperature. The handbooks give the value at 0 C:

(0 C) = 13.5951 gm/cm³

with negligible error. If you have one cubic centimeter of mercury at 0 C and heat it up to some temperature t, its volume increases by V cubic centimeters. If the inital volume (one cubic centimeter) is V₀, then the increase in volume is:

V = V₀ t

Here is called the coefficient of volume expansion, whose handbook value, with negligible error, is:

= 0.181 x 10^-3 deg^-1

Thus you can calculate the volume of mercury whose volume a 0 C was 1 cm³. Its mass remains 13.5951 grams, so you may then calculate the density at temperature t.

Calculate the value and error of 3/2 pV in joules. Theoretical physics predicts that this is the total kinetic energy of the air molecules in your small sample of gas.

Compare this energy to the amount of energy necessary to boil a gram of water from room temperature.

A Possible Extension

There is no experiment in this laboratory that can not be extended. We have an open invitation for all students to go beyond the experiments suggested in the Guide Sheets for extra credit. Consult with your Demonstrator whenever any experiment seems interesting enough that you wish to go further.

Here we mention one of many possible extensions for this particular apparatus.

When you are decreasing the volume of the gas you are doing work on it. Similarly, when you are increasing its volume you are doing negative work on it.

If done quickly, there is no time for energy to be exchanged between the gas and its surroundings; this is called adiabatic. Thus the temperature of the gas should rise when it is being compressed, and fall when it is being expanded.

Since the temperature is not constant, Boyle's Law should not be true in this circumstance.

Being careful not to spill the mercury, you can quickly change the volume of the gas and immediately read the volume and pressure. You can then determined the relationship between p and V.

Preparatory Questions

These questions should be answered and turned in to your Demonstrator before beginning the experiment.

Note:

These questions are intended to guide you in your preparation for the experiment. They do not have any "tricks."

1. Above you looked at an animation showing the effect of changing the height of the mercury reservoir on the heights of the mercury in the tubes. The distance between the heights of the mercury in the two tubes was shown with an arrow. The arrow changed color and intensity as the animation progressed. The color and intensity of the arrow is related to the pressure of the gas in the closed end of the tube with respect to atmospheric pressure. How does the color and intensity of the arrow represent this pressure?
2. This question refers to the figure to the right. Write down the equation for the pressure p at the point X situated a distance h below the surface of the liquid. Assume the density of the liquid is . Hint: consider the force that a column of liquid of cross sectional area A exerts at the point X. Then divide by A to get the pressure.
3. Write down two physical properties of real gases that might cause their behavior to deviate from Boyle's Law.
4. Given a fixed quantity of gas, will these deviations of the "ideal" behavoir of Boyle' s Law be smallest at large or small volumes?
5. The error in the pressure p is p, and the error in the volume V is V.
   • What is the error in 1/p?
   • What is the error in 1/V?
6. The error in the pressure p is p, and the error in the volume V is V. What is the error in the product p × V?

Summary

As promised, a summary of this page has been prepared. It is in pdf format and has a file size of 25k. You may access the summary by clicking on the red button to the right. It will appear in a separate window.

Authors

Earlier versions of this Guide Sheet have been worked on by David M. Harrison (1983), Joe Vise (1988), Tony Key (1995), Ruxandra Serbanescu (2001) and others.

This web version is by David M. Harrison in April 2003.

This is $Revision: 0.5 $, $Date: 2003/11/08 11:02:30 $ (y/m/d UTC).